Ostwald Ripening in Multiple-Bubble Nuclei

When you uncork a bottle of champagne, you will observe nuclei of bubbles and coarsening of them (Ostwald ripening). Ostwald ripening of bubbles is also observed in a power-generating turbine. While Ostwald ripening of bubbles is common and important in our life, this is highly non-trivial problem, since the interaction between bubbles is much stronger than that in other systems involving coarsening.

Fig.1: A snapshot of simulation. Larger bubbles become larger at the expense of smaller ones. (by H. Inaoka)

A mathematical framework describing coarsening processes was developed by Lifshitz and Slyozov, which was followed by Wagner. Now the theory is called LSW theory [1,2]. While the theory successfully explains the behaviors of coarsening processes for various systems, it is highly non-trivial whether the theory works for bubble systems. Especially, the mean-field treatment assumed in the theory should be verified for bubble systems, since the interactions between bubbles via the ambient liquid are much stronger than those in other systems, such as alloy, droplets, etc. Therefore, we perform molecular dynamics simulations of the cavitation process in order to study the validity of the LSW theory in homogeneous nuclei of bubbles.

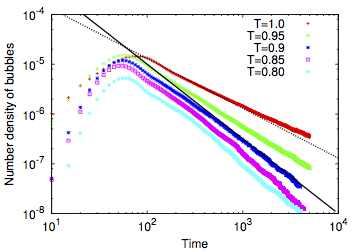
Fig.2: Temperature dependence of the scaling exponent. A total number of bubbles behaves as t^-x with x = 3/2 in low temperatures and x = 1 at high temperatures.

In order to investigate the coarsening process of bubbles from the molecular levels, a huge number of particles are required. Recent development in computational power, however, enables us to treat hundreds of millions of particles. We perform molecular dynamics simulations involving 700 million particles on 4,000 processors on the K computer, which is the largest computer in Japan. We observe the behavior of the total number of bubbles and find a crossover from the interface-limited (Wagner limit) to the diffusion-limited process (Lifshitz-Slyozov limit) as temperature increases. In both regimes, the exponents of the time evolution are well predicted by the theory. Our findings imply that the time scales between the relaxation time of the pressure in the ambient liquid and the coarsening rates are clearly separated, and therefore, the mean-field treatment is well justified.

（by H. Watanabe）

Reference

[1] I. Lifshitz and V. Slyozov, J. Phys. Chem. Solids 19, 35 (1961).
[2] C. Wagner, Z. Elektrochem. 65, 581 (1961).
[3] H. Watanabe, M. Suzuki, H. Inaoka, and N. Ito, J. Chem. Phys. 141 234703 (2014).

Press release by AIP publishing: How the Physics of Champagne and Soda Bubbles May Help Address the World’s Future Energy Needs