Bose-Einstein Condensation (BEC)
BEC is characteristic to quantum many body systems. In BEC, quantum character, which affects only microscopic physics at finite temperature, exhibit itself in macroscopic properties of matter. An old and most prominent example is Helium 4. Superconductivity may also be regarded as a special case of BEC in that two electrons are bound to form a quasi particle (Cooper pair) which obeys Bose statistics.
Macroscopic Wave Function
In condensed state, wave functions of many particles have the same phase. This makes it possible to express the whole many body state as a single macroscopic wave function that evolves with a equation similar to the Schroedinger equation. (Ref. “Finite-temperature transition in a quasi-2D Bose gas trapped in the harmonic potential“) Particles in this state are characterized only by phase and amplitude, and cannot behave independently. This suppresses entropy, leading to time-evolution without dissipation, such as superfluidity and superconductivity.
Ultracold Atoms
In old examples of BEC, such as superfluidity of Helium 4, the system is dense and interactions among atoms are strong. A typical text book example of BEC in dilute gas was observed rather recently (Nobel prize in 2001), which followed development of laser cooling techniques (Nobel prize in 1997). This is a close-to-ideal system and quantitatively accurate comparison between computer simulation and experiment is now possible.
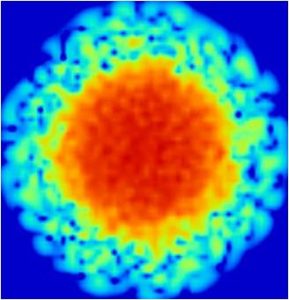
(by T. Sato)
Optical Lattice
By using standing wave formed by multiple laser beams, periodic potential can be created for ultra-cold atoms. Atoms trapped in such an artificial lattice (optical lattice) can be regarded as “an ideal solid”. Many research activities are going on recently. The principal motivation is possibility of observation of various physical phenomena (e.g., Bloch oscillation, Anderson localization, Mott transition (Ref. “Sharp peaks in the momentum distribution of bosons in optical lattices in the normal state“), etc) that were theoretically predicted but have been impossible to observed experimentally or only indirectly detected. In addition, possibility of using the optical lattice system as a quantum simulator and a basis for quantum computer stimulates research activities in this area even further.
Monte Carlo Method
However, such possibilities will come into reality only after we overcome a number of theoretical and technical difficulties. For a time being, we need to establish a quantitative correspondence between experiments and theory (model) by means of numerical simulation. To this end, we are developing new methods for quantum simulation of optical lattice systems and carrying out large scale computation on massively parallel supercomputers. (Ref. “Quantum Monte Carlo method for the Bose-Hubbard model with harmonic confining potential“, “Modification of directed-loop algorithm for continuous space simulation of Bosonic systems“)